In quantum mechanics, a quantum state describes an object on a tiny scale, typically representing the motion of particles. This state is mathematically represented by what is called a wave function. The states are just vectors which are elements in a vector space, known as the Hilbert space. Thus, vectors are well-known objects in linear algebra, making linear algebraic methods a powerful tool in quantum mechanics that seamlessly integrates geometry and algebra. With a solid grasp of the formulation and mathematical tools of quantum mechanics, several advanced problems in quantum science and technology can be reduced to more established and efficient methods in linear algebra.

One illustrative example is the transformation of a quantum circuit complexity problem into a geometry problem, which can then be studied through the lens of Riemannian geometry [1,2]. Quantum circuit complexity is a challenging issue in quantum computing, focusing on enhancing and advancing quantum algorithms to design efficient quantum circuit models. Here, quantum states transform into qubits, the carriers of quantum information, operated on by quantum gates within specialized algorithms. In this pursuit, the qubit replaces the classical bit of traditional computing.

Our work has successfully delved into the quantum circuit complexity within the framework of Riemannian geometry, particularly in the context of the three-qubit quantum Fourier transform (QFT) (Figure 1). We computed and proposed the Hamiltonian and Riemannian metric for the three-qubit QFT and formulated its geodesic equation in differential form. The resolution of this equation suggests that an efficient QFT of this kind can be realized.

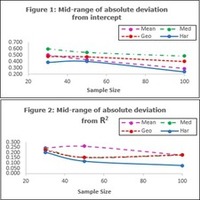
Additionally, we described quantum gates in a quantum circuit as elements of the SU(2) Lie group in operator representation and proposed a refined version of the three-qubit quantum Fourier transform within SU(3) (Figure 2). The frequency of the structure constant for the Lie algebra of su(8) in the Pauli basis is analyzed, confirming that all quantum circuits can essentially be generated using one-qubit gates and CNOT gates [3]. This provides insight into the relationship between the quantum circuit model and Lie Algebra.

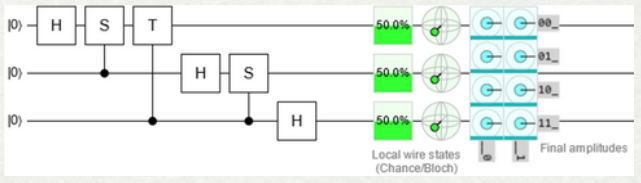
Figure 1: A quantum circuit of three-qubit quantum Fourier transform with Hadamard (H), S and T gate.

Figure 2: Matrix representation for corrected three-qubit quantum Fourier transform.

References

1. M.R. Dowling & M.A. Nielsen (2008). The geometry of quantum computation. Quantum Information & Computation, 8(10), 861–899.
2. H.E. Brandt (2009). Quantum computational geodesics. Journal of Modern Optics, 56(18-19), 2112–2117.
3. Chew Kang Ying, Nurisya Mohd Shah & Chan Kar Tim, (2022), Algebraic representation of three qubit quantum circuit problems, Malaysian Journal of Mathematical Sciences, 16(3):531-554.
   
   

About Author
Dr. Nurisya Mohd Shah
Department of Physics, Faculty of Science, Universiti Putra Malaysia
Expertise: Quantum Physics, Mathematical Physics, Noncommutative Quantum Mechanics
Email: risya@upm.edu.my

Date of Input: 18/03/2024 | Updated: 18/03/2024 | harithdaniel